Process performance index, is a statistical tool to verify if the sample that have generated from the process is capable to meet the customer requirement. It measures the performance of the process related to both dispersion and centeredness. In simple words, Ppk is an index of process performance, which tells how well a system is meeting specifications. And also how well the process is centered within the specification limits.
Ppk measurement is useful when you need to find out how much variation the process exhibits. Here, process performance is applied to a specific batch of material. It is used only when process control cannot be evaluated. Ppk tells you how the process has performed in the past. However, it cannot be used to predict the future, as the process is not in a state of control considering longer period.
Ppk calculations use sigma of the individual values. Process does not need to be centered on target value for this index as Ppk takes location of the process average in account. It penalizes the process by assuming that distances to the specification limits from the mean are equal to the smallest one. This does produce a desired result to maximize Ppk, which is to get suppliers to center their processes.
Interpreting Ppk
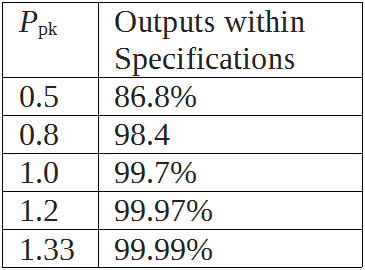
Larger the Ppk, lesser is the variation between process output and specifications. For instance, Machine A has Ppk of 1.0 and Machine B has Ppk of 0.5. From Ppk value, one can drive that Machine A is producing 99.73% of its output within specifications. Whereas, outputs produced from Machine B only 86.8% are within specifications. Therefore Machine A is better than machine B.
Ppk = [USL−μ/3σ,μi−LSL/3σi]
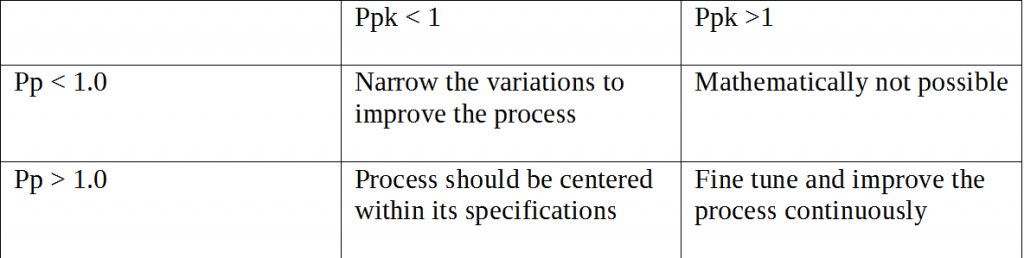
Where, Ppk should be used in conjunction with the Pp index. Ppk indicates what the process is doing and Pp indicates what it could do if it was centered. If they are not equal, some decision rules can be instituted indicating what action should be taken. Pp and Ppk will be equal if the process is centered in the specification. If they are not equal, the smaller the difference between these indices, the more centered the process is.
Some people tend to get confused between Ppk and Cpk, here is how they are different
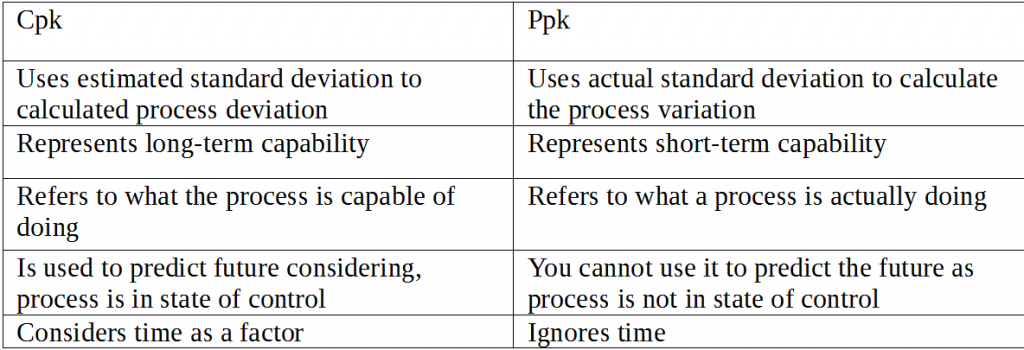
When the process is in statistical control the Ppk and Cpk values will converge. The reason being Sigma and the sample standard deviation will be identical. However, if the process is out of control, then the values will be distinctly different with a very wide margin.
